Ozette Triangle Trail 6 Ozette Triangle Trail 6?
Ozette Triangle Trail 6 Ozette Triangle Trail 6?
Monday, September 10, 2018.
Ozette Triangle Trail 16 Ozette Triangle Trail 16?
Ozette Triangle Trail 16 Ozette Triangle Trail 16?
Ozette Triangle Trail 7 Ozette Triangle Trail 7?
Ozette Triangle Trail 7 Ozette Triangle Trail 7?
Pages
Ozette Triangle Trail 49 Ozette Triangle Trail 49?
Ozette Triangle Trail 49 Ozette Triangle Trail 49?
Ozette Triangle Trail 9 Ozette Triangle Trail 9?
Ozette Triangle Trail 9 Ozette Triangle Trail 9?
Ozette Triangle Trail 25 Ozette Triangle Trail 25?
Ozette Triangle Trail 25 Ozette Triangle Trail 25?
Partitions of an equilateral triangle into equilateral: from 4 to infinity
It is very easy to split any equilateral triangle into 4 equal equilateral triangles, connecting the midpoints of its sides by segments, that is, drawing the middle mowing lines (Fig. 1, but).
Continuing to divide the resulting parts in the same way, we can divide the original triangle into 7, 10, 13. equilateral triangles, and in general, into any number of them like 3k 1 (where k. natural). Note that among the triangles, the partitions will necessarily be equal.
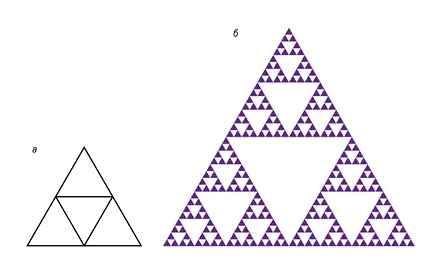
One of the self-similar figures is constructed similarly. Sierpinski triangle (such figures are called fractals). In an equilateral triangle, the middle mowing line is drawn and the middle of the four resulting triangles is “taken out”. This process is repeated in each of the other three triangles, etc., ad infinitum. The final figure (Fig. 1, b) has the same shape as its parts.
And if you divide the sides of an equilateral triangle not into 2 equal parts, but into 3, 4, etc.? Then you can split it into 9, 16.equal equilateral triangles (Fig. 2, but, b). After all, if you divide one of the sides into n equal parts, then the side of the small triangle will be in n times less than the side of the original, and then the area is in n 2 times less. This means that in the split there will be n 2 triangles. By the way, they could be counted by “layers”: in the upper layer. one triangle, in the next. 3, in the next. 5. in the lowest layer there will be 2n. 1 triangles. Along the way, we proved geometrically that 1 3 (2n. 1) = n 2.
Generalize to arbitrary triangles
All of the above can be easily generalized to the case of an arbitrary triangle by drawing three families of parallel lines (in each family, lines are parallel to one side and divide each of the other two sides by n equal parts). Now it is easy to understand how to split any triangle into n others like him, where n 5. Splitting into 6 triangles similar to the original one is obtained by making a drawing similar to Figure 2, but, and wipe off excess mowing lines (fig. 3, but). Splitting into 8 similar ones (fig. 3, b) is obtained from Figure 2, b, etc., for any even n, large 5. If n. odd, then after erasing you need to take one more step: divide the “upper” triangle with middle lines into four equal ones. Figure 3, in such a partition into 11 triangles is shown.
But not every triangle can be split into 2, 3 or 5 triangles similar to the original one.
Instead of a conclusion
It is not fully known which triangles are cut into 5 similar ones, see the article by B. Frenkin “On cutting a triangle into similar ones” (Kvant, 2008). For the development of the theme for polygons, see M. Gardner’s book “Mathematical Leisure” (Mir, 2000; ch. 24: “Dividing” figures on a plane).
Splitting into similar triangles
How to split a triangle into similar triangles? 1 How many triangles can be obtained with such partitions?
Rectangular triangles
Let’s find out which triangle can be split into two similar ones. Let the segment CD divides the triangle ABC into two similar ones: ACD and BCD. If a ∠ CAD = α, ∠ ACD = β, then ∠ BDC = α β (Fig. 4, but). Then in the triangle ACD there must be an angle α β, and it can only be an angle ADС. Means, ∠ АDС = ∠ ВDC = α β = 90 °. Then the original triangle is also right-angled, and ∠ ASB = 90 °.
Since α β = 90 °, then ∠ DCB = α, ∠ ABC = β, and triangles ACD and BCD like a triangle ABC (fig. 4, b).
Having drawn in any of the obtained triangles the height from the vertex D, we will break the triangle ABC into three triangles like him. Continuing this process, you can split a right-angled triangle into any number of similar ones. Is it possible to make these triangles equal? Sometimes you can.
So, if a right triangle ABC. also isosceles, height CD splits it into 2 equal right-angled isosceles triangles like ABC, and their heights drawn from the top D, give already 4. Continuing, we can split a right-angled isosceles triangle into 2 n equal triangles like it (n. any natural).
But this case is not the only one. Let the lengths of the legs of a right-angled triangle be integers m and k, then it can be broken down into m 2 k 2 equal triangles like it. To do this, draw the height from the vertex of the right angle and split one resulting triangle into m 2.and the other is on k 2 equal triangles, as in Figure 2. The resulting small right-angled triangles of two types are equal (in hypotenuse and acute angle) and are similar to the original one. Figure 5 shows an example of dividing a triangle with legs 5 and 7 into 74 = 5 2 7 2 equal triangles.
How to divide triangle into 5 equal parts?
Partitions into various similar triangles
And what triangle can be divided into triangles similar to it, among which there will be no equal? It turns out that any non-sided. Before explaining the solution, recall that in such triangles the ratios of the respective parties are equal. The generalized Thales theorem will help to construct the desired partition: parallel straight lines cut off proportional segments on the sides of the angle.
Consider a triangle ABC, in which BC / AC = k 1. Apply to the triangle ABC triangles 1, 2, 3, 4 and 5 We get a triangle divided into 6 unequal similar triangles.
Triangles ABC, 1, 2, 3, 4 are all different, since each next in k times more than the previous one.
But triangles 4 and 5 may turn out to be equal if k k 3 = k 4. Then we complete triangles 6 and 7, and replace triangle 5 with triangle 8. Triangles 7 and 8 are not equal, since k 6 ≠ k k 3 k 5. After all, if k k 3 = k 4.then k 6 = k 2 (k k 3) = k 3 k 5 3 k five.
Tasks for independent solution
Is it possible to split any triangle into three equal triangles, similar to the original?
Is it possible to break into five triangles, similar to the original, any: a) a right-angled triangle; b) (S. Markelov) non-rectangular triangle?
(T. Emelyanova) Cut a non-sided triangle into four similar triangles, among which not all are equal.
four. (A. Galochkin) A paper triangle with angles of 20 °, 20 °, 140 ° is cut along one of its bisectors into two triangles, one of which is also cut along the bisector, and so on. Is it possible after several cuts to get a triangle similar to the original?
five. (D. Shnol) Each of two similar triangles was cut into two triangles so that one of the resulting parts of the first is similar to one of the parts of the second. Are the remaining parts necessarily similar?
(M. Panov) Is it possible to split an equilateral triangle into 5 isosceles, but not similar in pairs?
1 Two triangles are similar if the angles of one are respectively equal to the angles of the other (the corresponding equality of the two angles is sufficient).
Cut a triangle into 4 triangles
Let us prove that property 2 implies property 3. Let a convex polygon F cut into parallelograms. It is necessary to prove that for any side of the polygon F there is another side parallel and equal to it. From each side of the polygon F a chain of parallelograms departs, that is, this side seems to move along them in parallel, and it can be split into several parts (Fig. 25.20). Since a convex polygon can have only one more side parallel to the given one, then all the branches of the chain abut on the same side, and its length is not less than the length of the side from which the chain exits. We can release a chain of parallelograms both from the first side to the second, and from the second to the first, so the lengths of these sides are equal.
It remains to prove that property 3 implies property 2. The method of cutting a polygon with equal and parallel opposite sides is shown in Fig. 25.21. After each such operation, we get a polygon with fewer sides, which still has property 3, and do the same with it until we come to a parallelogram.
25.23. Let’s use the result of the previous task. If the convex polygon M is cut into convex centrally symmetric polygons, then they can be cut into parallelograms. therefore M can be cut into parallelograms, i.e. M has a center of symmetry.
25.24. Let us prove by induction on n, that any 2n-a square whose sides are of the same length and opposite sides are parallel can be cut into rhombuses. For n = 2 this is obvious, and from Fig. 25.21 it is clear how the induction step is done.
25.25. Select in a regular octagon two mutually perpendicular pairs of opposite sides and consider, as in Problem 25.1, chains of parallelograms connecting opposite sides. There are rectangles at the intersections of these chains. Considering the other two pairs of opposite sides, we get at least one more rectangle. Parallelograms of each chain can be additionally cut so that the chain splits into several “tracks”, and in each track adjacent parallelograms would adjoin each other with whole sides, and not parts of sides. The union of the rectangles of the new partition is the same as the union of the rectangles of the original partition, so the proof is sufficient for the new partition. Each track has a constant width; hence, the length of one side of each rectangle included in the track is equal to the width of the track, and the sum of the lengths of all other sides is equal to the sum of all track widths corresponding to the second pair of sides. Consequently, the area of all rectangles included in one track is equal to the product of the track width by the length of the side of the polygon, i.e., it is numerically equal to the track width. Therefore, the area of all rectangles corresponding to two perpendicular pairs of opposite sides is 1, and the area of all rectangles in general is 2.
25.26. We denote the points of intersection of one of these lines with the others by A, B and C. For definiteness, we will assume that the point B lies between A and C. Let be D. intersection point of lines passing through A and C. Any straight line passing through a point B and does not pass through the point D, cuts the triangle ACD into a triangle and a quadrangle. 25.27. a) Let n straight lines divide the plane into an parts. Let’s draw one more straight line. In this case, the number of parts will increase by n 1, since the new line has n points of intersection with already drawn lines. therefore an 1 = an n 1. Since a1 = 2, then an = 2 2 3 ј n = (n 2 n 2) / 2. b) Having enclosed all the points of intersection of these lines in a circle, it is easy to check that the number of unbounded figures is 2n. Therefore, the number of limited figures is (n 2 n 2) / 2. 2n = (n 2. 3n 2) / 2.
25.29. Let’s call the straight line borderline for this figure, if it is a continuation of the line segment or ray that bounds this figure. It suffices to prove that the two figures under consideration cannot have more than four common boundary lines. If two figures have four common boundary lines, then one of the figures lies in area 1, and the other lies in area 2 (Fig. 25.24). The fifth boundary line of a figure lying in region 1 must intersect two adjacent sides of quadrilateral 1, but then it cannot be a boundary line for a figure lying in region 2.
25.30. Consider all the points of intersection of these lines. Let us prove that these points can lie on one side of at most two given lines. Suppose the intersection weights lie on one side of the three given lines. These lines form a triangle ABC. The fourth line cannot intersect only the sides of this triangle, that is, it intersects at least one extension of the side. For definiteness, let it intersect the extension of the side AB per point B at some point M. Then the points A and M lie on opposite sides of a straight line BC. A contradiction is obtained. Therefore, there are at least n. 2 straight lines, on either side of which are the intersection points. If we choose in the half-plane given by the straight line l, closest to l intersection point, then this point will be the vertex of the triangle adjacent to the straight line l. Thus, there is at least n. 2 lines, to which at least two triangles are adjacent, and two lines, to each of which at least one triangle is adjacent. Since each triangle is adjacent to exactly three straight lines, there are at least (2 (n. 2) 2) / 3.
25.31. If a P. the point of intersection of these lines, then from P comes out 2 l (P) segments or rays. In addition, each of x segments have two boundary points, and each of 2n rays has one boundary point. therefore
| 2x 2n = 2 | e | l (P) |
, i.e.
| x =. n | e | l (P) |
. 25.32. We carry out the proof by induction on n. For two lines, the statement is obvious. Suppose the statement is true for n. 1 straight lines, and consider a system consisting of n direct. Let be f. the number of parts into which the data n straight lines break the plane;
| g = 1 n | e | (l (P). one) |
. We discard one straight line from this system and for the resulting system of straight lines we define in a similar way the numbers f ў and g ў. If on the discarded line lies k points of intersection of straight lines, then f ў = f. k. 1 and
| g ў = 1 (n. one) | e | (l ў (P). one) |
. It is easy to check that
| e | (l (P). 1) =. k | e | (l ў (P). one) |
. By the induction hypothesis f ў = g ў. therefore f = f ў k 1 = g ў k 1 = g. It is also clear that the number of unbounded parts is 2n. 25.33. Let be ak ў. number of reds k-squares, a ў. the number of bounded red areas, the number of segments into which these lines are divided by their intersection points, is
| e | l (P). n |
(see problem 25.31). Each line segment is a side of at most one red polygon, therefore
| 3a ў Ј | e k i 3 | kak ў Ј | e | l (P). n |
, moreover, one inequality is attained if and only if there are no red k-squares where k 3, and another inequality is attained if and only if any segment is a side of the red k-gon, that is, any unbounded red area is an angle. The number of restricted areas is
| one. n | e | (l (P). 1) = c |
(see problem 25.32). therefore the quantity b ў bounded blue areas equals
| c. a ў i 1. n | e | (l (P). one). ( | e | l (P). n) / 3 = 1. (2n/ 3) | e | (2 l (P) / 3. 1) |
. Colors 2n unlimited areas alternate, so
| b = b ў n i 1 (n/ 3) | e | (2 l (P) / 3. 1) |
and
| a = a ў n Ј (2n | e | l (P)) / 3 |
, which means,
| 2b. a i 2 | e | (l (P). 2) |
.
25.39. It is clear that after n cuts will turn out n 1 piece. Since after each cutting the total number of vertices of the resulting figures increases by 2, 3 or 4, then after n cuts the total number of vertices does not exceed 4n 4. If after n cuts turned out to be 100 20-gons, then in addition to 20-gons there are also n 1. 100 pieces, since the total number of pieces is n 1. Since each piece has at least three vertices, the total number of vertices is at least 100 20 (n. 99) 3 = 1703 3n. Therefore, 1703 3n Ј 4n 4, i.e. n і 1699. It remains to prove that in 1699 cuts it is possible to cut a square as required. To cut a square into 100 rectangles, 99 cuts are enough, and to cut 16 triangles from each of these rectangles and turn them into 20-gons, 16,000 cuts are enough.
25.40. Let’s introduce a coordinate system with the origin at one of the vertices of the original rectangle and the axes directed along its sides. Cut the coordinate plane with straight lines x = n/ 2 and y = m/ 2, where m and n. integers, and color the resulting parts in a checkerboard pattern. If the sides of a rectangle are parallel to the coordinate axes, and the length of one of its sides is 1, then the sums of the areas of its white and black parts are equal. Indeed, with symmetry about the middle mowing line of the rectangle, the white parts turn into black ones and vice versa. For a rectangle with an integer side, a similar statement is true, because it can be cut into rectangles with side 1. It remains to prove that if the sums of the areas of the white and black parts are equal, then one of the sides of the rectangle is integer. Suppose both sides of the original rectangle are not whole. Direct x = m and y = n cut off rectangles from it, one of the sides of each of which is equal to 1, and a rectangle, both sides of which are less than 1. It is easy to check that in the last rectangle the sums of the areas of the white and black parts cannot be equal.
25.45. Among all the segments that cover the left end of the original segment, choose the one with the right end of the rightmost one, and denote this segment I1. After the segment is selected Ik, choose among all the segments that cover its right end, the one with the right end to the right. Thus, we will select several segments that completely cover the original segment. It remains to prove that the sum of their lengths does not exceed 2. The segment Ik 2 has no common points with Ik, because otherwise instead of Ik 1 we would have to choose Ik 2. Therefore, each point of the original segment of length 1 is covered by at most two segments Ik, i.e., the sum of the lengths of these segments does not exceed 2.
25.46. We will sequentially discard the segments covered by one or more of the remaining segments as long as possible. Let us direct the coordinate axis along this segment and denote the coordinates of the ends of the remaining segments by ak and bk (ak i ak 2. Two cases are possible. one. bk 1 Ј bk 2, then the segment numbered k 1 covered with numbered lines k and k 2. Obtained a contradiction.
bk 1 i bk 2, then the segment numbered k 2 is covered by a segment numbered k 1. Obtained a contradiction.
It remains to note that the sum of the lengths of either even or odd segments is at least 0.5.
25.47. Let be AB. the largest side of the pentagon. Consider the strip defined by the perpendiculars to the side AB, conducted through A and B. Since the corners EAB and ABC dumb, dots E and C lie outside this strip. Therefore the point D lies inside the strip, since otherwise the length of one of the segments ED and DC would be greater than the length of the segment AB. We denote the projection of the point D per segment AB through D1 (fig. 25.32). Then circles with diameters AD and BD completely cover the quadrangles AEDD1 and BCDDone.
Dividing a Triangle Into Fourths Using Medians : Triangles & Conversions in Math
25.48. a) Consider the largest square K cover and discard all squares that intersect with it. They lie inside a square whose side is 3 times the side K, therefore, the area occupied by them is not more than 81, where s. area K. Square K we refer to the selected ones and we will no longer consider it in the future. For the rest of the squares, do the same until all the squares are either selected or discarded. If the sum of the areas of the selected squares is S, then the total area of the discarded squares does not exceed 8S. Therefore, 1 Ј S eightS, i.e. S i 1/9.
b) Choose the circle with the largest radius, inflate it three times and discard all circles that lie entirely in this inflation. The remaining circles do not overlap with the first. Let’s do the same for them, and so on. The blow-ups of all the selected circles contain all these circles, and the blow-up area is 9 times the area of the original circle, so 9S i 1, where S. total area of all selected circles. Hence, S i 1/9.